Part 1 In The Series:
Overlooked Essentials in Geothermal Energy
Key Findings:
For every 1°C increase or decrease in the change in fluid temperature of an in-ground heat exchanger, the system may lose or gain the following amounts over a year:
400,000 kWh
ANNUAL ENERGY THAT MAY BE LOST OR GAINED PER °C CHANGE
$100,000
ANNUAL ENERGY COST THAT MAY BE LOST OR GAINED PER °C CHANGE
80,000 kg
ANNUAL CO2 THAT MAY BE EMITTED PER °C CHANGE
Introduction:
This post is part of our series on common practices in the geothermal industry which lead to changes in the geothermal system performance either during the pre-building process or after the system is installed. This post will help us quantify precisely how significant these choices are, and their impact on geothermal system efficiency, costs, and capacity.
This series will demonstrate how designers can more accurately characterize or quantify the longevity, peak capacity, and operating efficiency of all in-ground heat exchanging systems, including: shallow Ground Source Heat Pumps (GSHPs), conventional borehole GSHPs, foundation or pile GSHPs, underground thermal storage, and horizontal GSHPs.
In this article, we show how the system capacity can be doubled, and annual energy costs could be saved by up to $131,400 simply by changing the fluid temperature difference by 1°C.
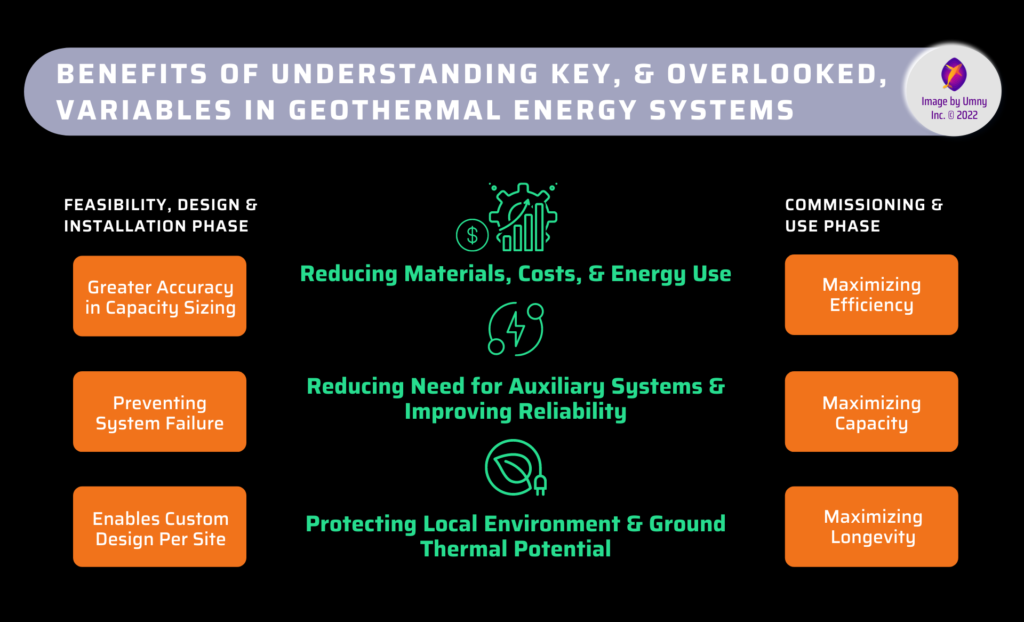
This post is an attempt to correct the common assumption that an error margin of +/-1°C or more in a geothermal system’s predicted or measured change in fluid temperature is “okay” or “not a problem,” or that losses to the fluid temperature of +/-1°C do not have a noticeable effect on the system.
This post is answering the question: What effect does a small change in fluid temperature (just 1°C) have on the performance of a geothermal system?

Understanding Geothermal Capacity:
To quantify the effect, we will use the Energy Equation and the Efficiency Equation to translate the fluid temperatures to total In-Ground Heat Exchange Capacity and the geothermal System Capacity as shown in Figure 1.
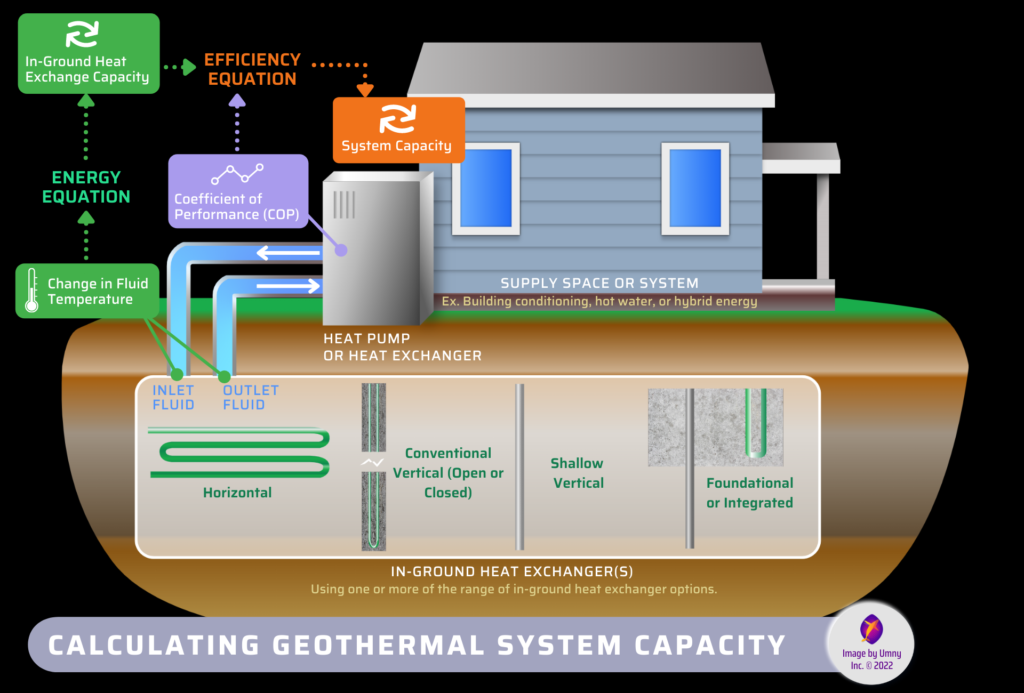
In this article we are exploring the effects of changing a geothermal system’s ΔT by plus or minus 1°C from any baseline ΔT.
What is ΔT?
ΔT is simply the temperature of the fluid ENTERING a heat exchanger minus the temperature of the same fluid LEAVING that same heat exchanger.
Change in Fluid Temperature = Tin – Tout = ΔT
Any geothermal system is essentially a large in-ground heat exchanger: fluid enters the geothermal pipes or piles, exchanges heat or cooling with the soil around the pipes or piles, and then leaves the ground at a different temperature. So, for the purposes of this article, ΔT can be thought of as:
ΔT = Fluid Temp. INTO Ground – Fluid Temp. OUT OF Ground
We want to be absolutely clear that when we talk about a change of 1°C in this article we do not mean that the ΔT is +/- 1°C in magnitude, we mean that the ΔT has changed from whatever value it was (it could have been -100°C, 20°C, or any other value) by 1°C.
So, if we think a geothermal system will produce a ΔT of 7°C, what is the significance if in reality it actually produces 6°C (-1°C change in ΔT) or if it produces 8°C (+1°C change in ΔT)?
Figure 2 is an example to illustrate what we are discussing in this article.
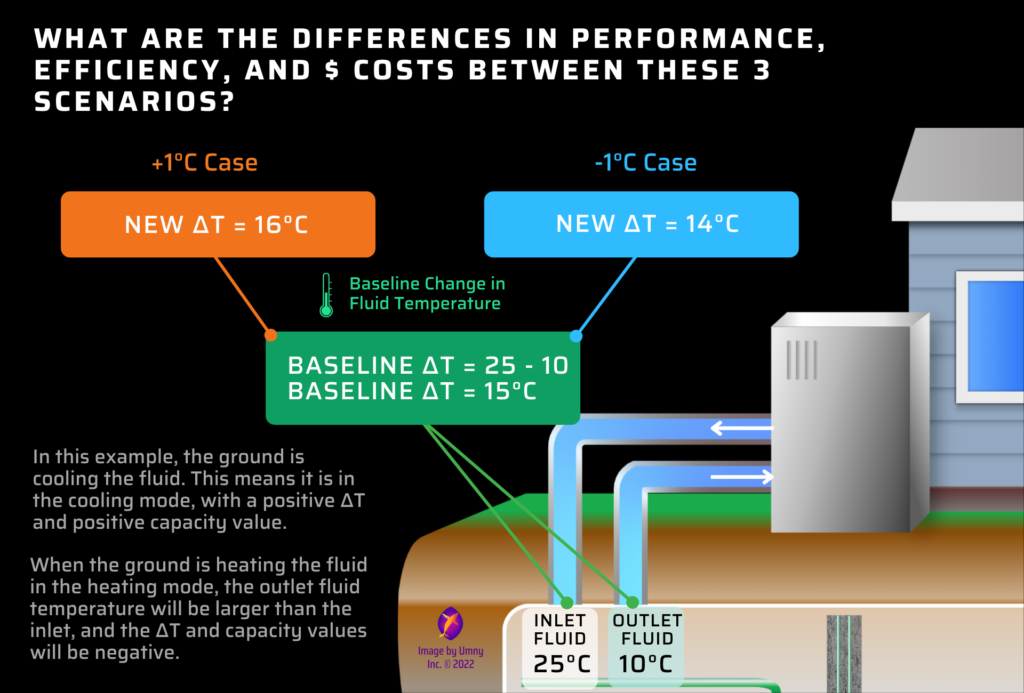
Why Are There “Negative” Capacities?
Due to the nature of the Energy Equation, values for capacity of these systems are negative in “heating mode” (when the geothermal system is moving heat from the ground and into the working fluid), and positive in “cooling mode” when the exchange cycle is reversed. Similarly, ΔT values are negative in heating mode (when the outlet fluid temperature is warmer than the inlet fluid temperature), and positive in cooling mode.
This change in sign simply shows the direction of heat flow, negative is heat flowing out of the ground, positive means heat is flowing into the surrounding ground. The capacity of the system is the magnitude of these values.
Quantifying the Effect on In-Ground Heat Exchange Capacity:
The central equation for determining the effect of a +/-1°C change in ΔT is the following Energy Equation, which describes the energy output of a geothermal system in terms of the fluid, flow rate, and change in fluid temperatures:

The In-Ground Heat Exchange Capacity is the amount of thermal energy in Watts that is exchanged across the in-ground component(s) at a given time – this can be thought of as the instantaneous capacity of the system.
Using the above equation, we can characterize the effect of a 1°C temperature change in the ΔT variable, in W, as being roughly 4166 * the Fluid Flow Rate, assuming that the fluid has a similar density and specific heat to water.

For Example:
Imagine a small residential geothermal system (providing heating/cooling) with a flow rate of 0.0001 m3/s and a ΔT of 0°C , the simplified equation becomes:

If the fluid temperature change being produced by the in-ground heat exchanger(s) increases by 1°C then A = 1. The result is that the system is producing 0.4166 W more cooling (or 0.4166 W less heating). If the fluid temperature change being produced decreases by 1°C, then A = -1, and the system is producing 0.4166 W more heat (or less cooling).
Significance In Terms of % of Total In-Ground System Performance:
How significant, then, is a 1°C change in the ΔT of a geothermal system? Like always, it depends. As designers and researchers, we need to be looking at the worst-case scenarios in order to protect our clients, and the environment, so let’s look at a few examples to illustrate the potential impact of a simple 1°C temperature change in the system.
There are many heating/cooling situations where the difference between the fluid inlet and outlet temperature (the ΔT) in the geothermal system is quite low, it is even possible for there to be NO difference between the inlet and outlet temperature, a ΔT of zero.
But if we were to take, for example, a system producing an outlet fluid temperature that is 2°C different than its inlet fluid temperature (which is not an uncommon situation in the real world) than a 1°C change in the outlet temperature can either reduce or increase the performance of the system by 50%, depending on the direction (+/-) of the temperature change and whether the system is supposed to be cooling or heating at the time.
To illustrate this point, six cases of ΔT changes are shown in Graph 1 below. This graph plots the In-Ground Heat Exchange Capacity of a “Baseline” system, whose ΔT matches the values on the x-axis ranging from -10°C to 10°C. Then, the two cases of +/-1°C change in ΔT are compared, to show the effects on heat exchange capacity. These calculations are performed using a high flow rate of 0.01 m3/s.
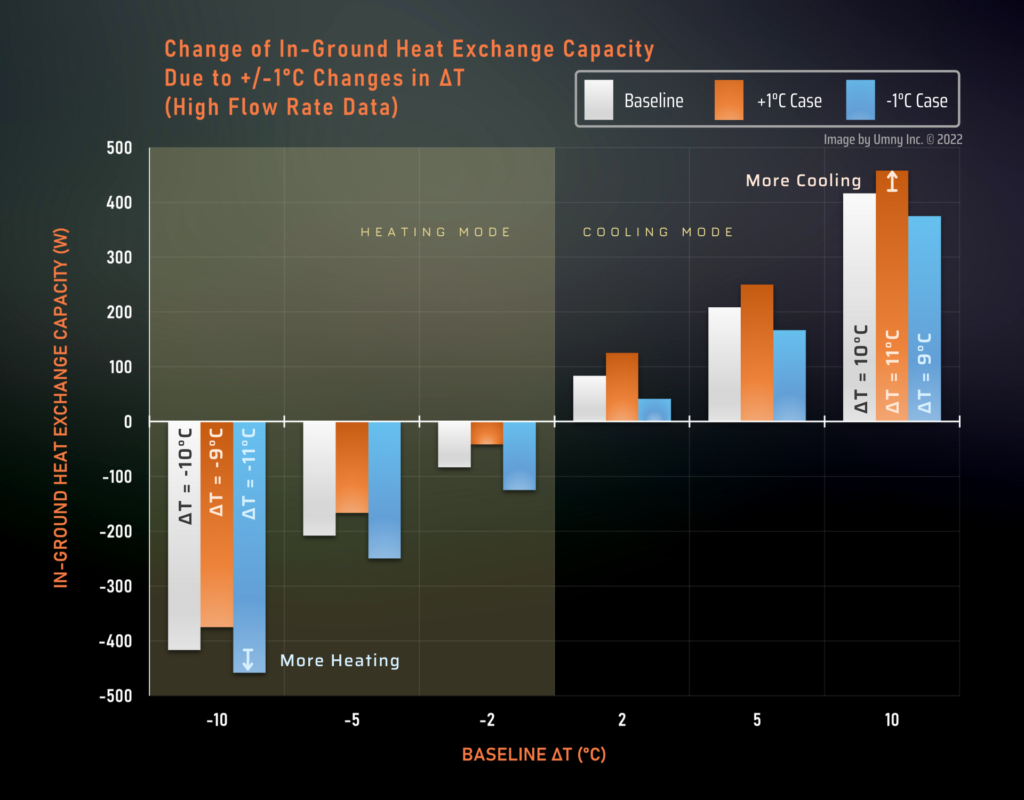
For all cases, as the ΔT increases in magnitude, the capacity also increases. The “+1°C Case” shows the new capacities that would be achieved by increasing the Baseline ΔT by 1°C and the “-1°C Case” shows the new capacities that would be achieved by decreasing the Baseline ΔT by 1°C.
When the ΔT is reduced by 1°C, the system can provide more heating in the heating mode than the baseline case. Similarly, when the ΔT is increased by 1°C then the system provides more cooling capacity in the cooling mode (see section on “Negative Capacities” above).
The total magnitude of the system’s capacity is dependent on the flow rate of the fluid in the system, but the changes in capacity due to changes in ΔT (in terms of %) are the same regardless of flow rate, as shown in Graph 2.

When comparing the percentage change in capacity due to +/- 1°C change in Baseline ΔT, we see that the effects are more significant (here up to 50%) for systems operating with a small change in fluid temperature (Baseline ΔT of +/- 2°C). Due to the total increase in capacity at the higher ΔT of 10°C and -10°C, increasing or decreasing this value by 1°C had a 10% effect on the heating/cooling capacity of the in-ground heat exchanger(s).
Generalizing the Performance Differences:
We can see from the above chart that there is actually a simple relationship we can use to determine the effect of a +/- 1°C outlet temperature change:
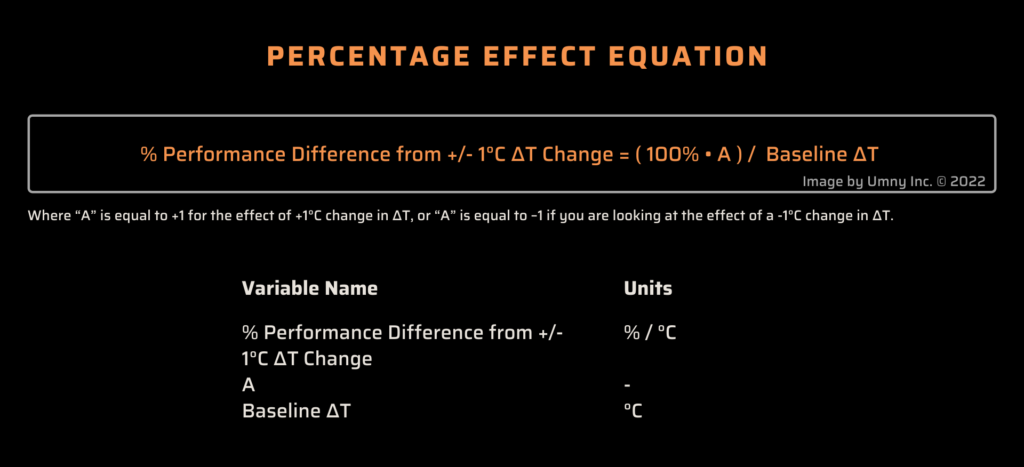
For relatively shallow geothermal systems, a 2°C difference between inlet and outlet is common and a 10°C fluid temperature difference is quite large.
For these systems, the effect of a small change in ΔT of only 1°C could improve or reduce the In-Ground Heat Exchange Capacity by 10 – 50%!
However, for more conventional, and deeper, geothermal systems which could have baseline temperature differences of 20 – 30 °C, the relative effect of a 1°C change in the output is reduced to only 2-5% of the total system output.
Quantifying the Effect on a Ground-Source Heat Pump:
Using the Efficiency Equation typical for Ground-Source Heat Pump (GSHP) systems, we can determine what effects the change in fluid temperature (and resulting change in In-Ground Heat Exchange Capacity) will have on the whole system performance (System Capacity) after this heat exchange is transferred through the heat pump to its target supply space or system. A similar method can be used to determine the resulting capacity through another form of heat exchanger by using the efficiency of heat exchange across those systems, typically given by the manufacturer.
System Capacity = Actual Energy Supplied by the Heat Pump
Using the Efficiency Equation, the actual supplied System Capacity can be calculated from the In-Ground Heat Exchange Capacity using the Coefficient of Performance (COP) of the system. Note that the efficiency in the cooling mode is commonly referred to as “EER”, but for our purposes we are describing the efficiency in both modes as COP.
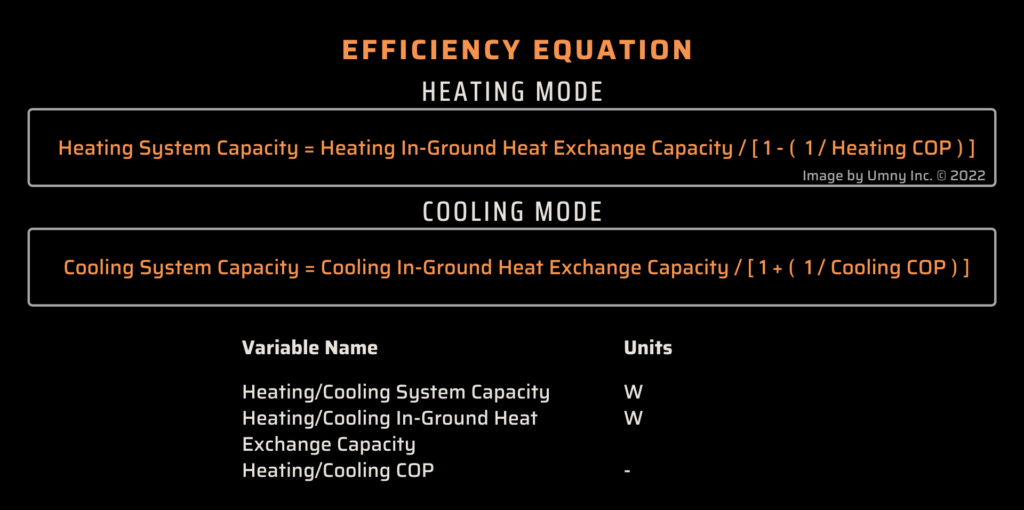
In this way, we can determine exactly how much energy is transferred from the ground through the heat pump and into the target supply area or system using the COP of the heat pump. To find the COP values, we rely on the accuracy of heat pump manufacturer specifications. In each heat pump manual, there is data relating the COP to the temperature of the fluid entering the heat pump from the ground portion of the system. In the schematic we’ve shown above (Figure 1), the COP of the heat pump is a function of the temperature of the outlet fluid from the in-ground heat exchanger(s).
Case Study with A Chosen Heat Pump:
Taking as an example, the Versatec Variable Speed 2-6 Ton Heat Pump manual, using the 072 – Full Load – Performance Data table at 21.0 GPM. We can determine the relationship between heat pump efficiency and outlet fluid temperature from the ground (or “entering water temperature” to the heat pump), by using a simple quadratic relationship from the points given in the heat pump manual, as shown in Graph 3.
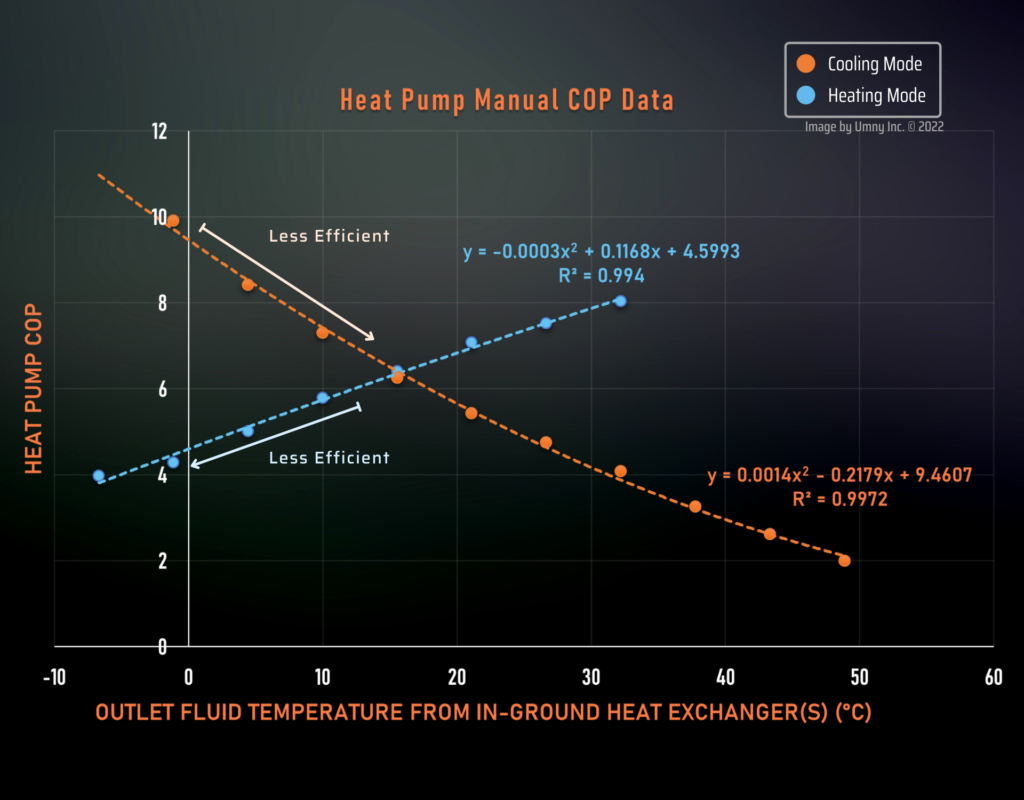
This graph shows that the heat pump’s efficiency in the heating mode reduces as the outlet fluid temperature from the ground reduces. However, the cooling efficiency reduces as the outlet fluid increases in temperature.
An error margin of +/-1°C in the fluid temperature prediction can move the system out of the operating range, or result in freezing of the fluid.
If the system is operating near the edge of a Heat Pump’s operating range, than the effect of a +/-1°C temperature change can be extremely dramatic. For example, it can cause damage to the equipment, it can make the system produce heating when you want cooling, or vice versa. Likewise if the system is operating near the fluid’s freezing point a drop of just 1°C can cause the fluid to begin to freeze.
This means that in some cases, a +/-1°C change in ΔT, can result in the complete failure of the geothermal system!
This change in ΔT has two effects on the System Capacity through the heat pump: first it results in a change in In-Ground Heat Exchange Capacity (discussed in the earlier section), and secondly, it results in a change in Outlet Fluid Temperature (assuming the inlet is constant) which affects the COP value (as seen in Graph 3) and may put the heat pump at risk for operating outside of the desired fluid temperature range.
Using the Efficiency Equation, and the COP relationship for this example heat pump, Graph 4 plots the System Capacity for six Baseline ΔT cases as well as their resulting Baseline COP. It then compares the changes to capacity and efficiency that occur when the ΔT is increased (orange case) and decreased (blue case) from the baseline by +/-1°C. For all cases, the inlet fluid temperature in the heating mode is constant at 5°C, and in the cooling mode at 25°C with a high flow rate of 0.01 m3/s.
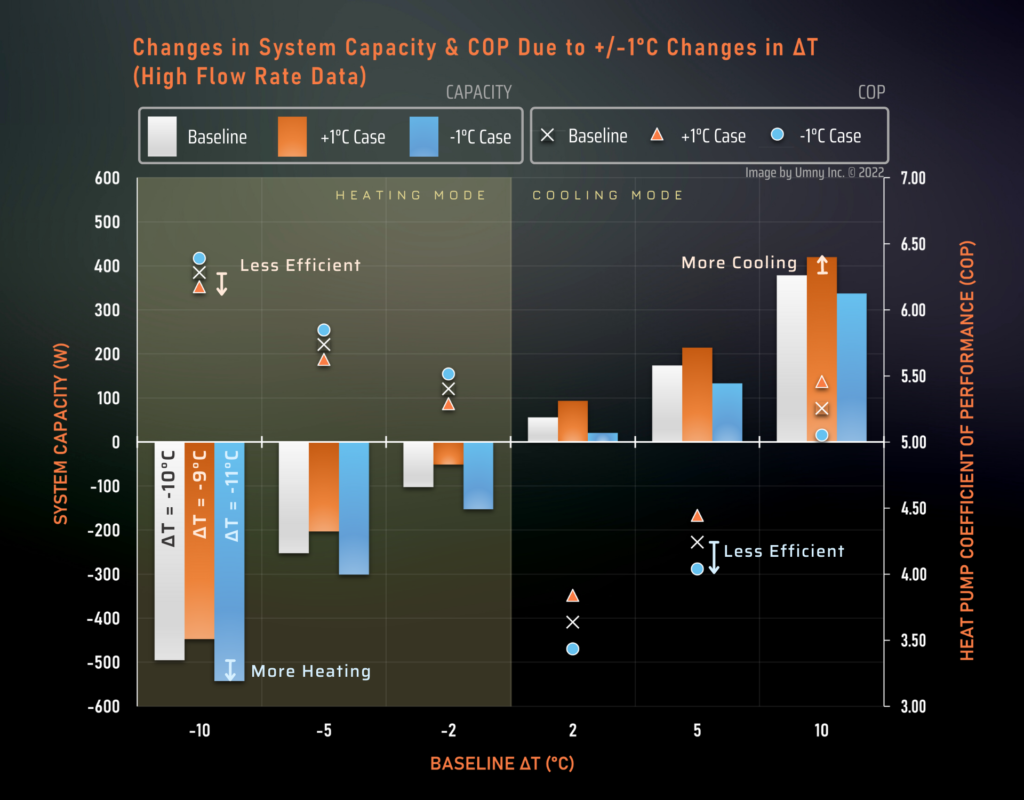
Note: A constant inlet temperature of 25°C was used for all cooling mode calculations, and 5°C for all heating mode calculations. The flow rate used was a high flow of 0.01 m3/s. These calculations are performed using the COP data from the Versatec Variable Speed 2-6 Ton Heat Pump manual, using the 072 – Full Load – Performance Data table at 21.0 GPM.
As with the In-Ground Heat Exchange Capacity, the System Capacity also increases with increasing magnitude of ΔT. For the heating mode, the change in baseline ΔT by -1°C resulted in more heating capacity from the heat pump and at a slightly higher COP. In the cooling mode, increasing the baseline ΔT by +1°C resulted in higher cooling capacity and higher efficiency (COP).
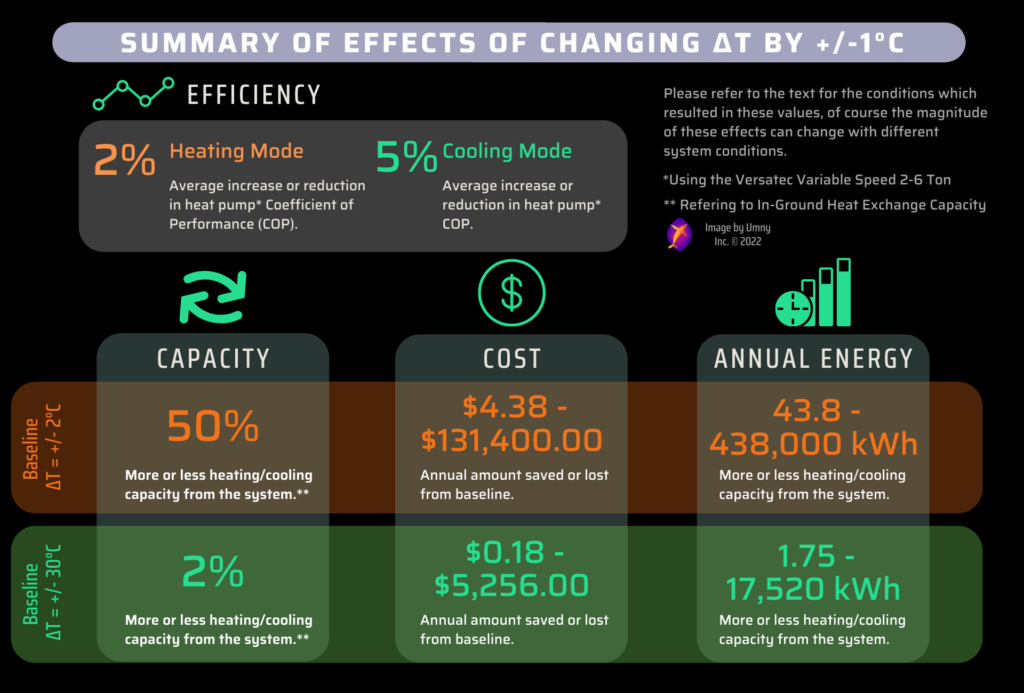
For the heat pump and operating conditions used in this case study, the COP value in the heating mode are, on average, 2% less (+1°C Case) or more (-1°C Case) than the baseline. For cooling mode, the heat pump is, on average, 5% more and less efficient for the +1°C Case and -1°C Case respectively.
The percentage differences in System Capacity due to a 1°C increase in ΔT ranged from –10% to –50% in the heating mode, and +11% to +69% in the cooling mode. Similarly, reducing ΔT by just 1°C changed the System Capacity for heating +10% to +49%, and –11% to –63% for cooling.
This means that simply by increasing the ΔT by 1°C, this heat pump may be able to produce 69% more cooling capacity.
What Effect Does This Have on Annual Energy Use (kWh) and Cost?
Until this point, we have been focusing on the instantaneous performance changes, but how do these effects add up over the course of a year of operation? And what is the resulting cost or savings from a +/-1°C change in ΔT?
To quantify these effects in Kilowatt-hours (kWh) produced by a geothermal system, or to put a cost amount on that change, we need to know how much energy the system is roughly producing. Table 1 breaks down the costs/gains in terms of thermal energy kWh and dollars for several different scenarios:
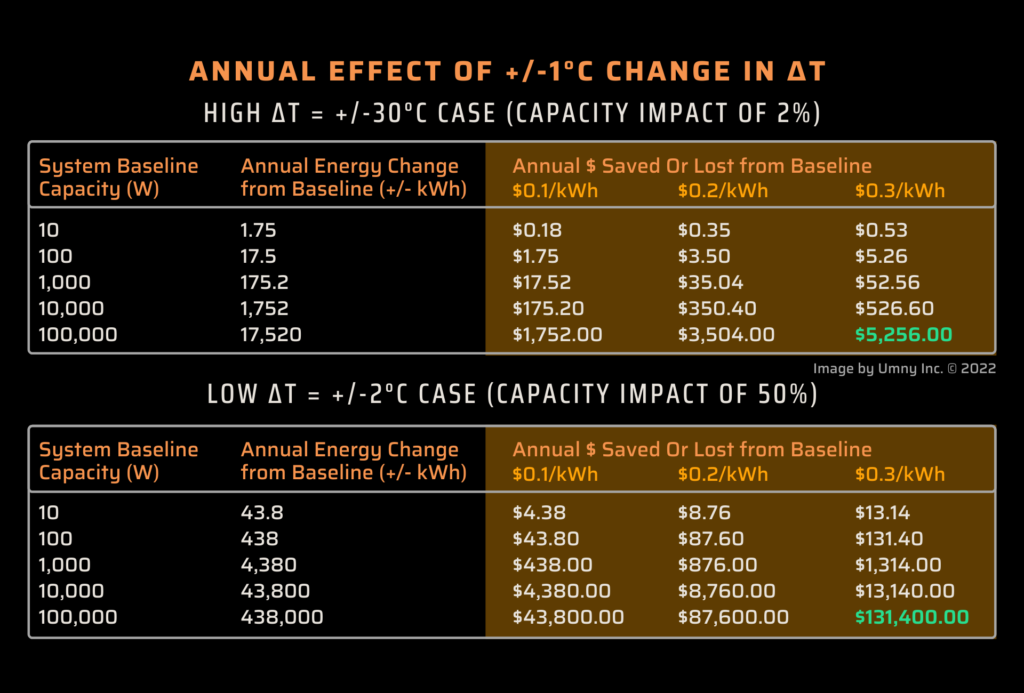
For a system with only 10 W of capacity, a change in ΔT of +/-1°C will have a small effect regardless of whether the Baseline ΔT is low or high – with the costs represented here, this would only result in a change of yearly energy cost between $0.18 to $13.14.
However, for larger systems, such as a 100 kW (or 100,000 W) capacity case, the resulting change in energy cost is between $1,752.00 to $131,400.00 just by increasing or decreasing the ΔT by 1°C!
What Effect Does This Have on Annual CO2 Emissions?
Using a simple conversion of the annual energy that is saved or lost from the above section, we can estimate the amount of annual CO2 emissions which could also be saved or lost.
With a natural gas conversion rate of 0.185 kg of CO2e per kWh, the maximum energy value of 438,000 kWh yields 81,030 kg of CO2 emissions per year, per 1°C change in Baseline ΔT.
Conclusion:
The significance of a 1°C change in the ΔT (change in fluid temperature) of a geothermal system depends on the size of that system and the typical differences between the inlet and outlet fluid temperatures in that system.
Geothermal systems, and Ground Source Heat Pumps, are particularly sensitive to small changes to fluid temperatures. For this reason we designed an AI software for one of our clients to predict the actual fluid temperature that an existing or proposed geothermal system might experience (read more about the Geo-Pile Predictive Software project here).
Generally, systems that are shallower and/or typically have small temperature differences between the inlet and outlet fluids will be much more significantly affected by a 1°C change in the outlet. In many realistic situations in this category, a 1°C ΔT change can increase or reduce the whole geothermal system’s performance by more than 50%! This can translate to hundreds of thousands of kWh of energy and hundreds of thousands of dollars being gained or lost each year, depending on the size of the system in question.
This article was written with only a +/-1°C change so that you can take the effects described here and scale them to the actual value that your system might have. For example, if you’re wondering what the impact of a sensor with a +/-0.5°C error margin is, the results here could be halved and this may still be an acceptable range for your system. However, when considering choices that result in higher changes to the designed Baseline ΔT (such as an uninsulated pipe), these impacts will be multiplied and become even more significant.
In conclusion, a seemingly small difference of 1°C in the fluid temperatures (ΔT) of a geothermal system, can have a massive impact on the performance, efficiency and profitability of that system.
Disclaimer
This blog is for educational purposes only. We are researchers with backgrounds in this field, and we are hoping to improve the quality, efficiency, and performance of geo-exchange and geothermal systems everywhere by contributing this science communication openly and freely to the public.